1983 | I Ching Index
Alternating between Complementary Conditions
for sustainable dialogue, vision, conference, policy, network, community and lifestyle
-- / --
Commentary B
on anexercise in metaphorical interpretation of the Chinese
Book of Changes
Original version (on networking
with references) published in Transnational Associations, 1983, 5, pp
245-258;
also published in Encyclopedia of
World Problems and Human Potential, 1994-5, vol 2, pp. 559-565
Alternation
Challenge of representation
Interpretation problems
Elaboration of a circular sequence
Transformation cycles
Circular representation: inner structure
Elaboration of a spherical map
Comment
1. Alternation
The vital point that emerges from the Chinese perspective of the previous note is that it is not sufficient to conceive of organizational conditions in isolation, as is the prevalent tendency among Western networkers. The processes of change in which a policy cycle is embedded, or to which it responds, require that the policy cycle consider itself in a state of transience within a set of potential conditions. It courts disaster if it attempts to "stick" to one condition such as "peace". If the dynamics of problem networks are not being contained by present strategies, as would appear to be the case, then organizational self-satisfaction is a recipe for the disaster-prone or the ineffectual. It creates a false sense of security. Any condition may be right temporarily, none is right permanently. The situation is somewhat analogous to many team ball games where if a player tries to retain the ball it will be taken from him by the opposing side, or else the team is penalized. Furthermore policy cycles opposing the "team" of world problems find themselves like novices having to deal with an opponent which handles the ball with a dynamism such as that of the Harlem Globetrotters or a shell-game con-artist. The focus shifts continually and is often where it is least to be expected in order to take advantage of weaknesses.
A policy cycle must continually "alternate" its stance within the network of transformation pathways in order to "keep on the ball" and "keep its act together". As with a surfer, a wind sailor, or a sailor on a rocking boat, if it fails to change its stance it will be destabilized, according to the I Ching, by one of 64 changing conditions through which it is forced to move in a turbulent environment.
The developmental goal can then be conceived as somehow lying "through" the exit of this labyrinth of traps for the unwary. More satisfactorily, it is perhaps "in" the art of moving through these conditions as progressively clarifying the locus of a common point of reference undefined by any of them (cf, the Sanskrit phrase "Neti Neti", roughly translated as "not this, not that"). It is this art which is extolled in describing the use of the I Ching or of Eastern board games. A similar notion has recently emerged from theoretical physics through the work of David Bohm (1980). He stresses the nature of an underlying "holomovement" from which particularities are successively "unfolded" once again. The significance is more readily apparent in the case of "resonance hybrids" mentioned earlier.
The problem for a policy cycle, an organization, an intentional community, a meeting, or even an individual, is then how to "network the alternation pathways together" and how to "alternate through a transformative policy cycle". Given that understanding of alternation seems only to be well-developed at the instinctual or sub-conscious level (eg walking, breathing, sex, dancing), the nature of alternation processes is explored in the Encyclopedia of World Problems and Human Potential (Section MZ). Extending the earlier metaphor of the "semantic piano" however, the challenge for policy cycles is then not simply to try to activate people by monotonous playing of single notes (eg "peace", "liberation", "development"), as presently tends to be the case. It is rather to acquire a perspective enabling them to collaborate in improvising exciting, rippling tunes with such notes (each of which might be I Ching condition) in order to bring out all the musical possibilities of alternation as explored in harmony, counterpoint, discord and rhythm.
In this sense the true potential of "policy cycling" lies in the transformational possibilities of "playing" on such instruments. Such an approach could perhaps provide the "requisite variety" by which the world problematique may be tamed, without breaking the spirit it embodies. A related challenge is then how to represent or map these transformation pathways in a memorable manner so that the range of possibilities becomes clear. In the Book of Changes a mnemonic system for the 64 conditions is given on the basis of 8 natural features of which people have both a instinctive and a poetic understanding. The features used as metaphors include: mountain, lake, wind, thunder, fight, ravine, earth and sky. Arguments in favour of some such topographically based mnemonic system are given in an earlier paper: "The territory construed as a map" (Judge, 1983). Such features contribute significantly to dissemination ofunderstanding about relationships between such conditions in contrast to the restriction of interest in such matters in the West to scientific elites. The Eastern board games mentioned above are deliberately used for educational purposes, whereas very few in the West have access to the computer simulation exercises with an equivalent orientation.
The following remarks, and those in the following notes, indicate some possibilities for producing an adequate general map of the transformation pathways are discussed.
2. Challenge of representation
The challenge for any organization is then to learn how to "alternate" through such a policy cycle rather than get trapped in any particular condition. To facilitate the response to this challenge, ways must be found to map this set of transformation pathways so that it becomes comprehensible as a whole that can be consciously negotiated. Some mapping possibilities are discussed below.
3. Elaboration of a circular sequence
Helmut Wilhelm reports that in the Sung period (960-1127) of Confucianism the scholar Shao Yung produced a tabular representation of the I Ching elements. This "table" was also represented as a circle which he reproduces. It was Shao Yung 's scheme which so excited Leibniz in the course of his reflections on the binary system.
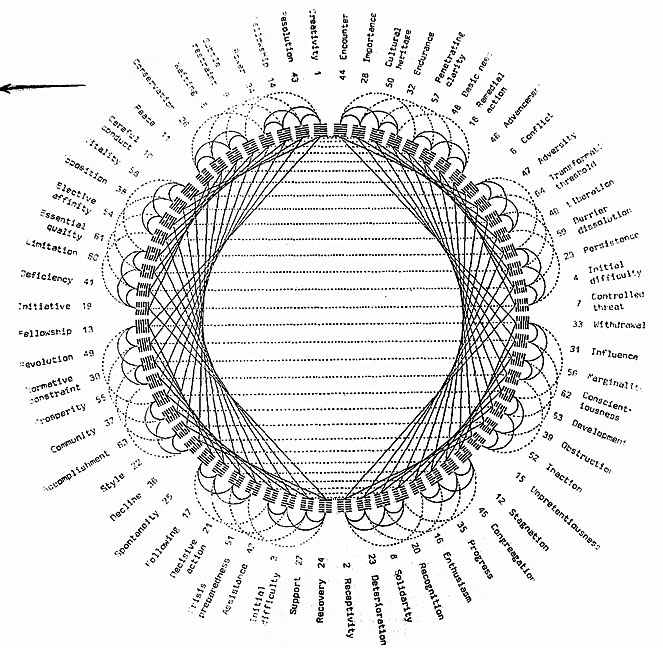
In this traditional representation the transformation pathways are implicit except for the circular sequence itself. It is however possible to render them explicit by simple adding them to the representation. One way of doing this results in a diagram such as Figure 1. The only lines added are for the six "high probability" transformation pathways associated with the six sub-conditions of each of the 64 conditions, as described in Section TP.
| Figure 1 - Map of transformations between global, 'heads-together' networking conditions ('top-in') | Figure 2 - Map of transformations between local, 'back-to-back' networking conditions ('top-out') | ||||||||||||||||||||||||||||||||
The conditions are denoted by hexagrams in a traditional circular order (each facing its negative image). The 6 transformations shown interlinking these conditions are those described in the accompanying text (in which only one line of each hexagram code is modified; see Figure 5 for multiple line modifications). The hexagram code is read here with the top line closest to the centre (in contrast to Figure 2). thus determining the condition numbers added. Note that a 7th transformation from each condition is that to its negative across the circle; an 8th is to its inversion, in the equivalent position in Figure 2. |
The hexagram codes appear here in the same order as in Figure 1. but because each code is read here with the bottom line closest to the centre (in contrast to Figure 1 ). the codes represent different numbered conditions in many cases. Only conditions 1,2, 27, 28, 29, 30, 61 and 62 do not change position. |
||||||||||||||||||||||||||||||||
 |
 |
||||||||||||||||||||||||||||||||
Before commenting further on Figure 1, some basic points must be made about the traditional circular sequence. It is made up of 64 distinct "hexagrams". The hexagram is the traditional Chinese way of representing a change condition by a binary code of 6 broken or unbroken lines (which can be considered identical to the binary bit-code used in modern computers). But there are at least two fundamental points about any such code, as pointed out in the case of computers by Xavier Sallantin (1975):
- there must be agreement as to what represents "broken" (or "on"), as opposed to "unbroken" (or "off"), or else the code may be mis-read as its own "negative";
- there must be agreement as to how the hexagram (or computer bit sequence) should be read, whether up-to-down (or right-to-left) or down-to- up (or left-to-right), or else the code may be mis-read in an "inverted" form. The traditional circular sequence does not distinguish between these two possibilities.
The second point as applied to Figure 1 means that in relating the 64 condition names to their traditional hexagram representations a decision has to be taken as to the direction in which a hexagram is to be read. In Figure 1 the decision has been made to read the hexagrams with the "top" of each towards the centre and the numbered conditions have been allocated accordingly. This means that there is an alternative interpretation, Figure 2, in which the bottom of each is towards the centre. Note that the order of the numbered conditions is then quite different. The pattern of transformation pathways remains the same, although the sub-conditions to which they relate are now different. The 3 transformation pathways for each hexagram that were originally indicated inside the circle in Figure 1 are indicated by the lines outside the circle in Figure 2.
Figure 3 - Transformation sequence through conditions in numerical order using Figure 1 hexagram positions |
Figure 4 - Transformation sequence through conditions in numerical order using Figure 2 hexagram positions |
| Odd-to-even transformations indicated by unbroken lines. | Odd-to-even transformations indicated by unbroken lines. The hexagrams bracketed together around the circumference are those described as denoting the 20 basic amino acids in the genetic code (34). In the Figure 1 order, these are denoted by the long transformation lines (5th sub-condition). |
 |
 |
4. Interpretation problems
The diagrams give rise to three problems:
(a) First problem: Either Figure 1 or Figure 2 can thus be considered as a very compact map of the 384 high probability transformation pathways. But the existence of two different and seemingly conflicting maps is obviously cause for reflection.
With regard to this problem, the existence of two interpretations can be explained as due to the manner in which the I Ching perspective is grounded on alternation between perspectives rather than being tied arbitrarily to one perspective. If two interpretations are possible there is necessarily an alternation between them according to the Chinese perspective. What then could the alternation between such contrasting interpretations signify? From the significance traditionally attached to the top and bottom of the I Ching hexagrams, it could be argued that in the case of organizations the two contrasting interpretations could relate to an inward global worldview alternating with an outward local worldview. The top-in perspective (Figure 1) would then correspond to a map of consciously interrelated contrasting perspectives on the wholeness in which they are embedded, signalled to some extent by the process whereby leaders of a group "put their heads together" and "share their views". The "enemy" is recognized as being within the group ("he is us"). The alternative top-out perspective would then correspond to a map of unexplicated solidarity in response to the challenges of the immediately perceived external environment, signalled to some extent by the process whereby group members "stand back-to-back" to face an external "enemy" as he manifests differently to each. To survive the group must to some extent alternate between these contextual and particular worldviews, rather as an individual alternates between right and left-brain perspectives. Lama Govinda (1981) notes that hexagrams are read from bottom-to-top to represent the sub-conditions of individual life, in contrast to the top-to-bottom direction for more fundamental or universal transformation.
(b) Second problem: Also of concern in their non-evident relation to the numbered sequence of conditions, which itself constitutes a single transformation cycle. This lack of relationship is especially evident when lines are traced between the conditions in that traditional sequence, as in the case of Figure 3 (using the Figure 1 order) or Figure 4 (using the Figure 2 order).
With regard to this problem, using Figure 3 or 4, inspection will show that the continuing alternation between "global inwardness" and "local outwardness" forces every second hexagram in the numbered sequence into its opposite form (eg 3 in Figure 1 becomes 4 in Figure 2; 5 becomes 6; etc) and back again. Only the hexagrams 1, 2, 27, 28, 29, 30, 61 and 62 are not "driven" through the numbered sequence by this alternation process (which here acts in a manner reminiscent of the effects of current alternation in the coil windings of an electric motor). The map is a map of alternation dynamics and cannot be appropriately understood as a conventional map of static structural elements.
(c) Third problem: In addition, other than the striking elegance of the pattern, it is not obvious why either the order of Figure 1 or 2 should be the basis for an appropriate map.
With regard to this problem, the "logic" of the circular representation is that every condition denoted by a hexagram is counterbalanced by its "opposite" across the circle. In effect the broken lines are converted into unbroken lines and vice versa (thus partially containing the variations in significance of broken and unbroken lines noted above). In addition to the six high probability transformations from (and to) each condition, there is therefore a seventh transformation through the numbered sequence (by inversion of the code reading direction) and an eighth transformation into its opposite (through "negative" code bits of a hexagram acquiring a "positive" connotation and vice versa).
Given the striking relationship already noted by Schönberger between the I Ching 64-hexagram code and the genetic 64-codon code, the fundamental nature of the circular representation may also be illustrated by using it to map the 20 amino acids basic to biological organization. In Figure 1 these are denoted completely by the set of (long) transformation lines linking quarters of the circle. For example, according to Schönberger, asparagine is denoted by (the transformation between) the hexagram pair 34-43, the more complex amino acid threonin is denoted by (the symmetrically balanced transformation lines) 11:5:26:9, and the "stop" codes amber and ochre are denoted by the individual hexagrams 56 and 33 respectively. In the Figure 2 map the hexagrams denoting each amino acid, rather than being equidistant, are brought together side-by-side, as is illustrated around the circumference of Figure 4.Whether this suggests that certain well-defined transformation processes are as essential for the life of an organization or policy cycle as those 20 amino acids are for biological organization, is a question for further investigation.
5. Transformation cycles
A striking feature of Figure 1 (or 2) is the manner in which the transformation pathways of different types differentiate the circle so clearly into:
- (a) 2 halves of 32
- (b) 4 quarters of 16
- (c) 8 groups of 8
- (d) 16 groups of 4
- (e) 32 groups of 2
- (f) 64 groups of 1
In the light of current interest in the distinct functions of right and left brain perspectives, group (a) can be considered an interesting representation of the limited number of pathways linking such halves and the manner in which the halves are each separately integrated. In the light of Jungian investigation of the four basic psychological functions (sensation, feeling, intellect, intuition), group (b) can be considered an interesting representation of the transformation pathways by which these are linked and separately integrated as semi-independent functions. The 4 masculine and 4 feminine archetypal versions of these functions distinguished by psychoanalysts can in turn perhaps usefully be represented by group (c).
The question that now emerges is whether it is possible to elaborate some kind of typology of transformation "cycles" for organizations or policy cycles. Such a typology would clarify the different kinds of way that, for example, the two functional halves, or the four functional quarters are interlinked. For it is highly probable that organizations or policy cycles can "survive" by using the simplest possible transformation cycles that enable them to renew themselves, but that richer and more effective policy cycling is only possible when more complex transformation pathway cycles are used. It is therefore to be expected that some organizations only manage a 4-transformation cycle linking four functional quarters but are quite incapable of handling the subtler functional transformations. Many organizations probably get stuck in cyclic "traps" because they cannot enrich the transformative cycles on which they depend. In addition to what has been termed the "high probability" transformations, based on the modification of a single line in a hexagram denoting a policy cycle condition, some other transformations of lower probability are shown in Figure 5. These too may form part of transformation cycles.
|
Figure 5 - Map of selected complex transformations between network conditions |
| Using the same circular order as for Figures 1 to 4, transformations are indicated between hexagrams for cases where two lines of the hexagram code are modified (see Figure 1 and 2 for single line transformations). The transformations selected are for different combinations of the inner three lines of each code (since those for the outer three link neighbouring hexagrams in a pattern similar to that around the circumference Of Figure 1 and 2). Other combinations do not appear to result in significantly different patterns. The hexagram codes may be read either in terms of the Figure 1 ('top-in') or the Figure 2 ('top-out') orders from which the corresponding numbered conditions may be obtained. |
 |
6. Circular representation: inner structure
A different approach to circular representation forms part of the conclusion of an extensive study by the renowned Buddhist scholar Lama Anagarika Govinda in a recent book entitled: The Inner Structure of the I Ching: The Book of Transformations (1981). His preference for "transformation" in the title is to be compared with the conventional translation as "change".
The special interest of this study, in contrast to the many studies of I Ching commentaries, is that it focuses on the structure of the I Ching itself as a system of signs in which "two values were alternated and finally combined into eight symbols, which by replication yielded sixty-four hexagrams."
Lama Govinda concentrates on the problem of the relationship between two traditional representations of the set of transformations. The first is the "abstract order" of Fu Hi which essentially determines the order of balanced polarities from which Figure 1 and 2 were derived. The second is the "temporal order" of King Wen which emphasizes the developmental sequence of phenomena. In order to make the movements from one condition to another graphically visible the author concludes that it only seems possible to find a unifying principle in the Fu Hi system.
His detailed investigations lead him to propose Figure 6. This shows the position of all 64 I Ching conditions projected onto a circular diagram. A unique feature of his focus on the "inner structure" is that this diagram results from the interplay between the 8 fundamental conditions from which the 64 are derived. The 8 are each denoted by a half- hexagram, namely a trigram. Depending on the order in which any given pair of trigrams is read, one of twohexagrams is thus defined. It is the condition numbers of these alternatives which are indicated on the straight lines within the circle. Each line thus represents two transformative movements. The eight conditions around the circumference represent those cases when the two trigrams are identical. Thus the straight lines denote transformations governed by the relationship between the 8 fundamental conditions denoted by each doubled trigram on the circumference.
|
Figure 6 - Projection of all conditions
(hexagrams) onto a circle |
| In Figures 1 to 5 the transformations between conditions are indicated by lines and curves (whether broken or unbroken). In Figure 6 those transformations are all represented as occurring within the 8 points around the circumference, whereas the lines represent the dynamic conditions denoted by the individual hexagrams positioned in a circle in Figures 1 to 5. Each line in Figure 6 indicates two possible conditions of change (just as each line in Figures 1 to 5 indicates two possible directions of transformation). The order of the 8 points around the circumference of Figure 6 corresponds to the order of the same points around the circumference of Figure 2 ('top-out' interpretation). |
 |
What then is the relationship between Figure 6 and Figures 1 to 5? As noted above, in Figures 1 to 5 the circle of hexagrams may be split into eight parts in each of which the trigram on the inside is identical. One of the hexagrams in each part also has the outside trigram equal to the inside one. It is these eight (1, 2, 29, 30, 51, 52, 57 and 58) that are positioned around the circumference in the "top-out" order of Figures 2 and 4. Comparison with these Figures will show that the transformations from any numbered condition are here indicated by the lines (or points) to which it is connected through these fundamental positions, whether one or more hexagram lines are modified. In this sense Figure 6 is a much more compact representation than Figure 2 and 5. There is an intriguing resemblance between some of Lama Govinda's other diagrams of transformation between trigrams (represented by "curves" and "lines") and aspects of the structure of Figure 1 and 2. In graph theory terms, Figure 6 is a "dual" of Figures 2 and 5 combined, in that the transformation lines in the latter correspond to the transformation points in the former. Even in this representational convention there is advantage in alternating between both forms.
Also of great interest is Lama Govinda's very detailed investigation of sub-patterns of transformation connecting groups of 8 conditions traditionally called "houses". These patterns provide an important basis for any further investigation of the typology of transformation cycles called for above. It also enables him to clarify the relationship between the numerical sequence and the abstract order of Figure 6 by determining in Figure 7 the four symmetrical sub-patterns from which Figure 6 is constituted.
|
Figure 7 - Sub-patterns of networking
conditions extracted from Figure 6 |
|
The numbered sequence of 64 conditions is split into A groups in numerical order. The patterns for each group are shown in the relevant diagram as a part of Figure 6. This establishes a relationship between the numerical sequence and an abstract order (which is the basis for Figures 1 to 5). Note that the reconstruction of this arrangement is only possible as a result of recognition, from internal structural evidence, of the error noted below. N.B. In producing Figure 6 from the elements of Figure 7, Lama Govinda
concludes (4, pp. 145-147) with Richard Wilhelm (12),that the traditional
numerical order of the hexagrams in current works is slightly in error:
35 and 36 should replace 3 and 4; 21 and 22 should replace 35 and 36;
and 3 and 4 should be inserted between 56 and 57. |
|
 |
 |
| Network conditions 1 to 16 | Network conditions 17 to 32 |
 |
 |
| Network conditions 33 to 48 | Network conditions 49 to 64 |
7. Elaboration of a spherical map
One interesting approach to this is to consider how Figure 6 would be transformed if it were to correspond to the alternative "top-in" order of Figure 1 and 3, instead of the "top-out" order of Figure 2. In effect the square formed by conditions 51, 52, 57, 58 in Figure 6 is simply rotated about the axis of conditions 1, 2; Conditions 1, 2, 29 and 30 do not move. The new sequence around the circumference is then 1, 58, 29, 51, 2, 52, 30, 57, as in Figures 1 and 3. If conditions 1 and 2 are considered as fixed "poles", a continuous rotation between the fixed positions 29 and 30 may be seen as transforming the circular representation into a spheric one. This dynamic model would need to be interpreted in terms of lines of force, as in the analysis of an electric motor or dynamo.
For reasons discussed in earlier papers, there are advantages in seeking a representation whose completeness is highlighted by basing it on an approximation to a spheric surface. The question then becomes how to cut up that surface into 64 units which will be assumed firstly to take the form of regular areas and secondly to be of identical form. (Other approaches are of course worth exploring.) Since the 64 phases (hexagrams) result from a conceptual system based on an eightfold complexification of 8 fundamental phases of change (trigrams), the problem can initially be reduced to one of representing the latter on a spherical approximation. The simplest such polyhedral approximation is the regular octahedron with eight triangular facets (see Figure 8). In allocating the 8 phases to these facets it would obviously be advantageous to do so such that their three high probability transformation pathways are highlighted.
|
Figure 8 - Octahedron as basis for mapping 8 fundamental networking conditions onto a sphere |
Figure 9 - Eightfold subdivision of the triangular facet of an octahedron. |
| The 64 networking conditions are derived from 8 fundamental conditions (represented by the doubled hexagrams indicated on the circumference of Figure 6). Each of the 8 may be denoted by one triangular facet of the octahedron. The allocation of the conditions, and the transformational relationships between them, can then be mapped onto the geometry of the octahedron (as one of the simplest polyhedral approximations to a sphere). This is discussed in the inset (below). | In order to represent all 64 networking conditions on an octahedron (Figure 8), each triangular face can be sub-divided into 8 equal areas as shown. Some of the possible conventions concerning the allocations of sub-conditions to the triangle, and the transformational relationships between them, are discussed in the inset (below). |
 |
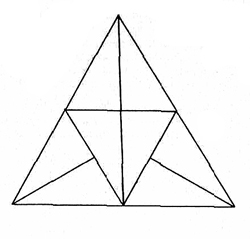 |
Returning to the 64 phases, the problem can now be defined as one of how to divide up each of the triangular facets of the octahedron into eight equal parts so that eight phases can be represented within each such triangle. This can be done as shown in Figure 9. In this way the 64 phases can each be given a unique location on a polyhedral structure which can be easily projected onto the surface of a sphere.
There remains the problem of how to order the eight phases within each facet in Figure 8 so that within the completed figure the six high probability transformation pathways of the 64 phases are highlighted. It would seem, as with the standard problem ofgeographical map projections onto a two-dimensional surface, that there are a number of approaches to be explored. Each would be based on a different convention and would lead to a different arrangement with different advantages.
8. Comment
The Book of Changes is recognized as striking a remarkable balance between logical, structural (left-brain) precision and intuitive, contextual (right-brain) nuances of comprehension. For 3,000 years it has proved to be a unique achievement in relating the qualitative to the quantitative in a manner which is both practical and poetically appealing -- qualities for any blueprint for a new world order.
In the exercise for Section TP, most of the poetic appeal has been sacrificed. It does demonstrate that it is possible to interpret the insights of an Eastern classic into the jargon of Western management, however much of a "profanation" this may appear to those who know the original. An important consequence of the elimination of metaphor (despite the argument of Section MZ) is the loss of vital mnemonic keys with which the original is replete with good reason. Much of value has therefore been lost, as in any interpretation, despite the seeming advantages to be gained from the precision of the alternative presentation. Clearly some of the distortion is due to the alternative framework, whilst much is due to the limitations of the interpreter. Other interpretations could strike a more graceful balance between jargon and insight.
The acid test is of course whether this interpretation is useful to the formulation of sustainable policy cycles. Is it possible to relate the conditions described to the practical issues to be encountered? Can policy-makers use or adapt the maps of transformation pathways reproduced here? The answers are for the future. But the precision of the framework of the Book of Changes, linking such contemporary topics as "development", "liberation", "peace", "revolution", with what have here been termed "basic need", "deficiency" and "cultural heritage", offers an intriguing challenge to reflection and comprehension. The topics recall many of the concerns of the Goals, Processes and Indicators of Development project (1978-82) of the United Nations University.
|
Figure 10 - Interrelationship of economic functions
in management systems |
 |
With regard to the important problem of representation, it is appropriate to note that schematic diagrams of similar form have already been produced in combining Eastern insights and a Western management emphasis. A striking example is that of Figure 10. Erich Jantsch (1980), in his wide-ranging synthesis of self-organizing systems and their implications for policy-making and human development, draws attention to metabolic transformation cycles such as the carbon cycle shown in Figure 11. Indeed, given the fundamental nature of the representation system and its relationship to the basic amino acids, it is worth investigating to what extent the set of interconnected metabolic cycles and pathways does not illustrate the kinds of transformation pathways which need to be identified for organizations. The map of metabolic pathways could prove to be a provocative challenge to organizational sociologists of the future.
| Figure 11 - Carbon cycle as a detail of metabolic pathways. |
 |
It is also tempting to see the 6 (+1) basic transformations from each condition (in Figure 1 and 2) in terms of catastrophe theory, as qualitative equivalents to the 7 characteristics kinds of catastrophe to which natural conditions are subject. The containment of plasma in fusion research suggest other insights concerning the containment of energy and the avoidance of "quenching".
This commentary began with a concern with how to reduce the drain of "energy" and significance from policies, organizations and meetings to which some of the transformation conditions respond. Is there not some possibility, like the search for the Holy Grail, that the challenge of giving form to sustainable policy cycles may be of equivalent complexity and form as that of containing plasma energy?
(Commentary C: Interrelating incompatible viewpoints)
Earlier version in 2nd edition of Encyclopedia of World Problems and Human Potential (1986)
|
For further updates on this site, subscribe here |


